Next: Numerical results of MHD Up: Numerical results for EAST Previous: Numerical results for EAST
The tokamak equilibrium used in this paper is reconstructed by EFIT code by
using the information of profiles measured in EAST experiment[9].
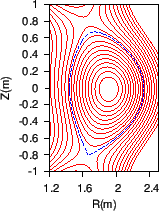
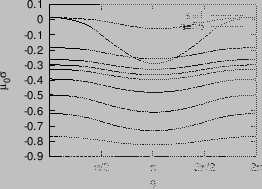
The shape of flux surfaces within the last-closed-flux surface (LCFS) are
plotted in Fig. 11, where
![]() curves are also
plotted. In the paper, I said that the equilibrium was a double-null
configuration with the LCFS connected to the lower X point. This is wrong. The
configuration with the LCFS connected to the lower X point should be called
lower single null configuration. The double-null configuration is a
configuration with LCFS connected to both the lower and upper X points. In
practice, if the spacial seperation between the flux surface connected to the
low X point and the flux surface connected to the upper X point,
curves are also
plotted. In the paper, I said that the equilibrium was a double-null
configuration with the LCFS connected to the lower X point. This is wrong. The
configuration with the LCFS connected to the lower X point should be called
lower single null configuration. The double-null configuration is a
configuration with LCFS connected to both the lower and upper X points. In
practice, if the spacial seperation between the flux surface connected to the
low X point and the flux surface connected to the upper X point,
![]() , is smaller than a value (e.g. 1cm), the configuration can be
considered as a double null configuration, where
, is smaller than a value (e.g. 1cm), the configuration can be
considered as a double null configuration, where
![]() is the spacial
separation between the two flux surfaces on the low-field side of the
midplane.
is the spacial
separation between the two flux surfaces on the low-field side of the
midplane.
  |
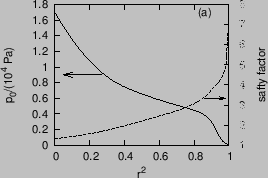
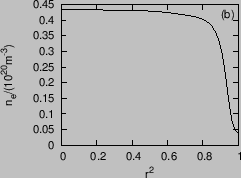
The profiles of safety factor, pressure, and electron number density are plotted in Fig. 12.
  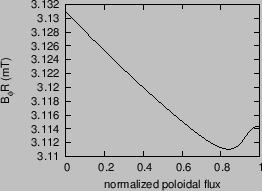 |
The mass density ![]() is calculated from
is calculated from
![]() , where
, where ![]() is the mass of the main ions (deuterium ions in this discharge),
is the mass of the main ions (deuterium ions in this discharge), ![]() is the
number density of the ions, which is inferred from
is the
number density of the ions, which is inferred from ![]() by using the neutral
condition
by using the neutral
condition ![]() (impurity ions are neglected).
(impurity ions are neglected).
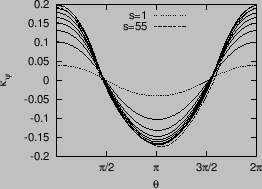 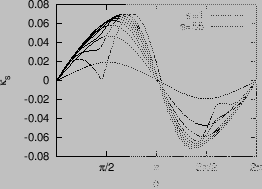 |
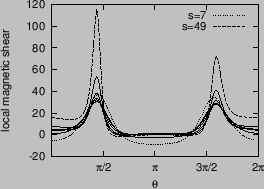 |
 |
yj 2015-09-04