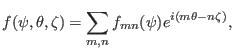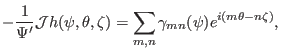The usefulness of the contravariant form [Eq. (250] of the magnetic
field lies in that it allows a simple form of
 operator in a coordinate system. (The operator
operator in a coordinate system. (The operator
 is
usually called magnetic differential operator.) In
is
usually called magnetic differential operator.) In
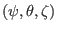 coordinate system, by using the contravariant form Eq. (250), the
operator is written as
coordinate system, by using the contravariant form Eq. (250), the
operator is written as
Next, consider the solution of the magnetic differential equation, which is
given by
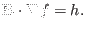 |
(256) |
where
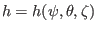 is some known function. Using Eq.
(255), the magnetic differential equation is written as
is some known function. Using Eq.
(255), the magnetic differential equation is written as
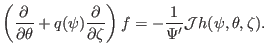 |
(257) |
Note that the coefficients before the two partial derivatives of the above
equation are all independent of  and
and 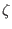 . This indicates that
different Fourier harmonics in
. This indicates that
different Fourier harmonics in  and
and 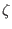 are decoupled. As a result
of this fact, if
are decoupled. As a result
of this fact, if 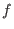 and the right-hand side of the above equation are Fourier
expanded respectively as
and the right-hand side of the above equation are Fourier
expanded respectively as
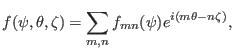 |
(258) |
(note that, following the convention adopted in tokamak
literature[6], the Fourier harmonics are chosen to be
 , instead of
, instead of
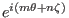 ), and
), and
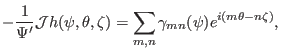 |
(259) |
then Eq. (257) can be readily solved to give
![$\displaystyle f_{m n} = \frac{\gamma_{m n}}{i [m - n q]} .$](img813.png) |
(260) |
The usefulness of the straight line magnetic coordinates
 lies in that, as mentioned in the above, it makes the coefficients
before the two partial derivatives both independent of
lies in that, as mentioned in the above, it makes the coefficients
before the two partial derivatives both independent of  and
and  ,
thus, allowing a simple solution to the magnetic differential equation.
,
thus, allowing a simple solution to the magnetic differential equation.
yj
2018-03-09
![]() operator in a coordinate system. (The operator
operator in a coordinate system. (The operator
![]() is
usually called magnetic differential operator.) In
is
usually called magnetic differential operator.) In
![]() coordinate system, by using the contravariant form Eq. (250), the
operator is written as
coordinate system, by using the contravariant form Eq. (250), the
operator is written as