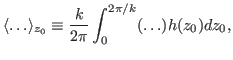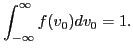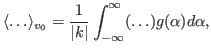Averaging over initial spatial location and
velocity of particles
Assume the distribution function of the particles is given by
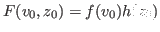 and
and
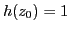 , i.e. the distribution is uniform in space.
, i.e. the distribution is uniform in space.
Consider the averaging over the initial position of particles. Define
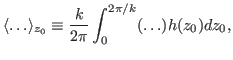 |
(25) |
which is an operator averaging over the initial position of particles in the
interval of one wave length. Using this operator on both sides of Eq.
(24), we obtain
Note that the term
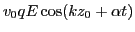 corresponds to the power
of the electric field acting on those particles that move at a constant speed
corresponds to the power
of the electric field acting on those particles that move at a constant speed
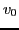 . Also note this term is reduced to zero when averaged over the initial
position
. Also note this term is reduced to zero when averaged over the initial
position 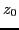 no mater whether it is a resonant particle (i.e.,
no mater whether it is a resonant particle (i.e.,
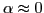 ) or not (i.e.,
) or not (i.e.,
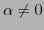 ). (This important fact is seldom
mentioned in textbooks, which is one of the motivations that I wrote this
note.) Changing to the new variable
). (This important fact is seldom
mentioned in textbooks, which is one of the motivations that I wrote this
note.) Changing to the new variable
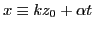 , the above
equation is written as
, the above
equation is written as
which agrees with Eq. (8) in Chapter 8 of Stix's book. Next, we will average
Eq. (29) over the distribution of initial velocity. Define the
averaging operator in velocity space
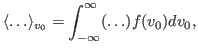 |
(30) |
where 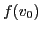 is the one-dimensional distribution function, which satisfies
the following normalizing condition
is the one-dimensional distribution function, which satisfies
the following normalizing condition
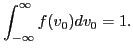 |
(31) |
Changing to the variables
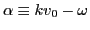 , equation
(30) is written as
, equation
(30) is written as
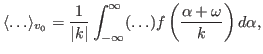 |
(32) |
Define
 |
(33) |
then equation (32) is written as
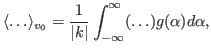 |
(34) |
Taking the average over the initial velocity, Eq. (29) is written
as
It can be proved that the integration
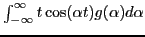 and
and
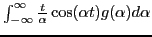 in the above equation approach zero rapidly
for large
in the above equation approach zero rapidly
for large 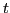 (refer to Sec. 2.5.3). Thus, in the sense of time
asymptotic, we are left with only the integration of the first term, which is
written as
(refer to Sec. 2.5.3). Thus, in the sense of time
asymptotic, we are left with only the integration of the first term, which is
written as
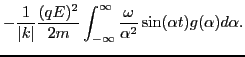 |
(36) |
yj
2016-01-26
![]() and
and
![]() , i.e. the distribution is uniform in space.
, i.e. the distribution is uniform in space.