Consider the form of matrix 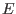 in the cylindrical geometry limit, in which
the equilibrium quantities are independent of poloidal angle. Equation
(
in the cylindrical geometry limit, in which
the equilibrium quantities are independent of poloidal angle. Equation
(![[*]](crossref.png) ) indicates that the geodesic curvature
) indicates that the geodesic curvature 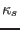 is zero in
this case. Thus, the matrix elements
is zero in
this case. Thus, the matrix elements 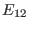 and
and 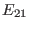 are zero. Next,
consider the matrix elements
are zero. Next,
consider the matrix elements 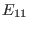 and
and 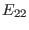 . Because all equilibrium
quantities are independent of the poloidal angle, different poloidal harmonics
of the perturbation are decoupled. Therefore, we can consider a perturbation
with a single poloidal mode number . For a poloidal harmonic with poloidal
mode number
. Because all equilibrium
quantities are independent of the poloidal angle, different poloidal harmonics
of the perturbation are decoupled. Therefore, we can consider a perturbation
with a single poloidal mode number . For a poloidal harmonic with poloidal
mode number 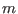 , matrix element
, matrix element 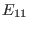 is written
is written
and matrix element 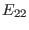 is written
is written
The continua are the roots of the equation
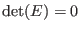 , which, in the
cylindrical geometry limit, reduces to
, which, in the
cylindrical geometry limit, reduces to
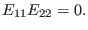 |
(218) |
Two branches of the roots of Eq. (218) are given by
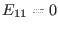 and
and
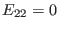 , respectively. The equation
, respectively. The equation
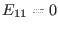 is written
is written
 |
(219) |
which gives
 |
(220) |
which is the Alfvén branch of the continua. Figure 1a plots
the results of Eq. (220). The equation
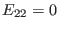 is written
is written
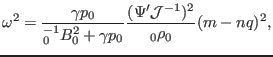 |
(221) |
which is the sound branch of the continua. Figure 1b plots the
results of Eq. (221).
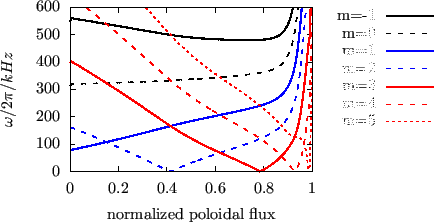
Figure:
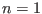 Alfven continua (left) and sound continua
(right) in the cylindrical geometry limit for
Alfven continua (left) and sound continua
(right) in the cylindrical geometry limit for
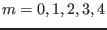 , and
, and 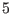 (calculated by using Eqs. (220) and (221)). The
equilibrium used for this calculation is for EAST discharge #38300@3.9s
(G-eqdsk filename g038300.03900, which was provided by Dr. Guoqiang Li). The
number density of ions is given in Fig. 12. Because the Jacobian
(calculated by using Eqs. (220) and (221)). The
equilibrium used for this calculation is for EAST discharge #38300@3.9s
(G-eqdsk filename g038300.03900, which was provided by Dr. Guoqiang Li). The
number density of ions is given in Fig. 12. Because the Jacobian
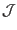 in toroidal geometry depends on the poloidal angle, the
average value of
in toroidal geometry depends on the poloidal angle, the
average value of
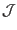 on a magnetic surface is used in evaluating
the right-hand side of (220).
on a magnetic surface is used in evaluating
the right-hand side of (220).
  |
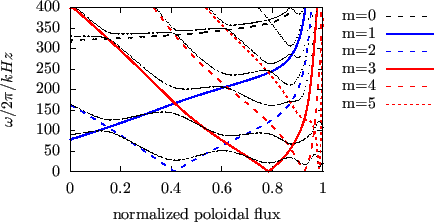
Figures compares the Alfven continua in the cylindrical limit with those in
the toroidal geometry. The results indicate that the Alfven continua in the
toroidal geometry reconnect, forming gaps near the locations where the Alfven
continua in the cylindrical limit intersect each other.
Figure 2:
Comparision of the the Alfven continua in toroidal geometry (black
solid lines) and Alfven continua in the cylindrical limit (other lines). The Alfven continua in toroidal geometry are obtained by using the
slow-sound-approximation. The equilibrium is EAST discharge #38300 at
3.9s.
 |
The result in Eq. (220) is not clear from the view of physics since
it involves the Jacobian, which is a mathematical factor due to the freedom in
the choice of coordinates. Next, we try to write the right-hand side of Eq.
(220) in more physical form. In cylindrical geometry limit, magnetic
surfaces are circular. Thus the radial coordinate can be chosen to be the
geometrical radius of the circular magnetic surface, and the usual poloidal
angle (i.e., equal-arc angle) can be used as the poloidal coordinate. Then the
poloidal magnetic flux is written as
 |
(222) |
where 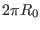 is the length of the cylinder. We know that
is the length of the cylinder. We know that 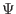 used in
the Grad-Shafranov equation is related to
used in
the Grad-Shafranov equation is related to  by
by
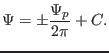 |
(223) |
Using Eqs. (222) and (223), we obtain
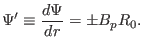 |
(224) |
Next, we calculate the Jacobian
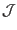 , which is defined by
, which is defined by
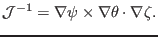 |
(225) |
Since we choose 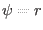 and
and
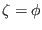 (the positive direction of
(the positive direction of
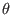 is count clockwise when observers view along the positive direction
of
is count clockwise when observers view along the positive direction
of 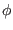 ), the above equation is written
), the above equation is written
Using Eqs. (224) and (226) ,
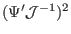 is
written
is
written
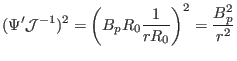 |
(227) |
Using these, Eq. (220) is written
 |
(228) |
Using the definition of safety factor in the cylindrical geometry
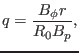 |
(229) |
equation (228) is written
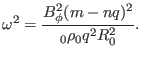 |
(230) |
In the cylindrical geometry, the parallel (to equilibrium magnetic field)
wave-number is given by
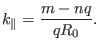 |
(231) |
Using this, Eq. (230) is written
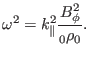 |
(232) |
Using the definition of Alfven speed
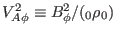 , the above equation is written as
, the above equation is written as
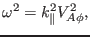 |
(233) |
which gives the well known Alfven resonance condition. For later use, define
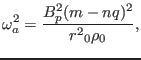 |
(234) |
then Eq. (228) is written as
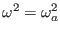 .
.
Similarly, by using Eq. (227), equation (217) for
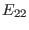 is written as
is written as
Then equation
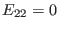 reduces to
reduces to
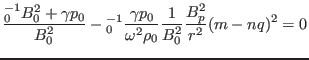 |
(235) |
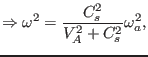 |
(236) |
where
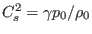 ,
,
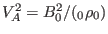 .
Equation (
.
Equation (![[*]](crossref.png) ) gives the sound branch of the continua. For present
tokamak plasma parameters,
) gives the sound branch of the continua. For present
tokamak plasma parameters, 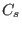 is usually one order smaller than
is usually one order smaller than 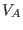 .
Thus, equation (236) indicates the sound continua are much smaller
than the Alfven continua for the same
.
Thus, equation (236) indicates the sound continua are much smaller
than the Alfven continua for the same 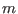 and
and 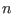 .
.
yj
2015-09-04
![]() in the cylindrical geometry limit, in which
the equilibrium quantities are independent of poloidal angle. Equation
(
in the cylindrical geometry limit, in which
the equilibrium quantities are independent of poloidal angle. Equation
(![[*]](crossref.png) ) indicates that the geodesic curvature
) indicates that the geodesic curvature ![]() is zero in
this case. Thus, the matrix elements
is zero in
this case. Thus, the matrix elements ![]() and
and ![]() are zero. Next,
consider the matrix elements
are zero. Next,
consider the matrix elements ![]() and
and ![]() . Because all equilibrium
quantities are independent of the poloidal angle, different poloidal harmonics
of the perturbation are decoupled. Therefore, we can consider a perturbation
with a single poloidal mode number . For a poloidal harmonic with poloidal
mode number
. Because all equilibrium
quantities are independent of the poloidal angle, different poloidal harmonics
of the perturbation are decoupled. Therefore, we can consider a perturbation
with a single poloidal mode number . For a poloidal harmonic with poloidal
mode number ![]() , matrix element
, matrix element ![]() is written
is written
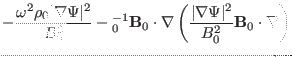
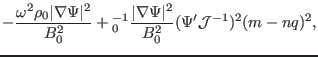



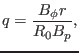
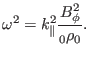
![]() is written as
is written as
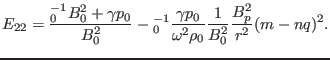
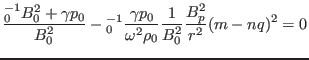
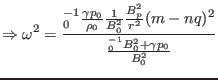
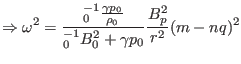
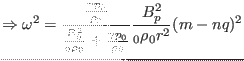
![[*]](crossref.png) ) gives the sound branch of the continua. For present
tokamak plasma parameters,
) gives the sound branch of the continua. For present
tokamak plasma parameters,