Figure 21 compares the 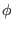 coordinate surface of
coordinate surface of
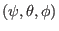 coordinates with the
coordinates with the  coordinate surface of
coordinate surface of
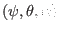 coordinates.
coordinates.
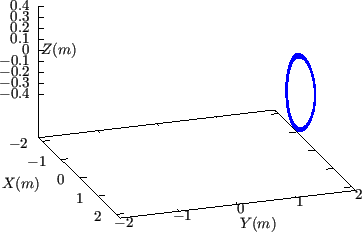
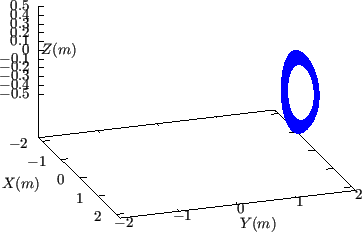
Figure 21:
Comparison between isosurface of
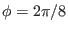 (projection of magnetic field lines onto
(projection of magnetic field lines onto
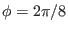 plane) and
isosurface of
plane) and
isosurface of
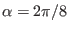 . The
. The  isosurface is made of a
family of contours of
isosurface is made of a
family of contours of
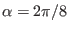 , which are all magnetic field
lines. These field lines are traced by starting from a series of points on
the low-field-side midplane
, which are all magnetic field
lines. These field lines are traced by starting from a series of points on
the low-field-side midplane
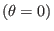 at different radial locations and
the field lines are followed by a complete poloidal loop. The radial range
is given by
at different radial locations and
the field lines are followed by a complete poloidal loop. The radial range
is given by
![$ \psi _N \in [0.4 : 0.5]$](img74.png) , where
, where 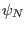 is the normalized
poloidal magnetic flux. Magnetic field from EAST discharge #59954@3.03s.
is the normalized
poloidal magnetic flux. Magnetic field from EAST discharge #59954@3.03s.
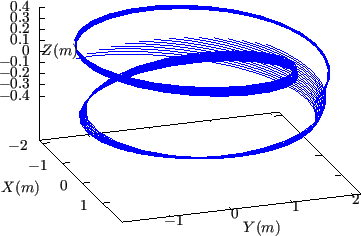 |
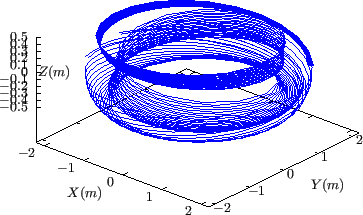
Figure 22:
The same plot as in Fig. 21 but with a larger radial
range.
![$ \psi _N \in [0.4 : 0.7]$](img76.png) , where
, where 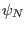 is the normalized poloidal
magnetic flux.
is the normalized poloidal
magnetic flux.
 |
yj
2018-03-09
![]() coordinate surface of
coordinate surface of
![]() coordinates with the
coordinates with the ![]() coordinate surface of
coordinate surface of
![]() coordinates.
coordinates.



