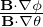 ∇ψ +
∇ψ +  ∇𝜃 +
∇𝜃 +  ∇ζ,
∇ζ,
In solving the MHD eigenmode equations in toroidal geometry, we also need the radial differential operator ∇ψ ⋅∇. Next, we derive the form of the operator in (ψ,𝜃,ζ) coordinates. Using
∇f = 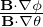 ∇ψ + ∇ψ +  ∇𝜃 + ∇𝜃 +  ∇ζ, ∇ζ,
|
the radial differential operator is written as
where ∂(qδ)∕∂ψ and q∂δ∕∂𝜃 are given respectively by Eqs. (259) and (253). Using the above formula, ∇ψ ⋅∇ζ is written as
![[ ]
∂(qδ) 2 ∂δ-
∇ ψ ⋅∇ζ = − ∂ψ |∇ ψ| + q∂𝜃∇ 𝜃⋅∇ ψ .](tokamak_equilibrium380x.png) | (291) |
This formula is used in GTAW code.