Integration in velocity space

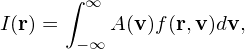
Consider a general velocity moment of the distribution function:

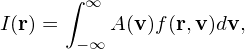∫ ∞ I(r) = A(v)f(r,v)dv, −∞

(34)

where A(v) is a known function. Using expression (33), the above moment is written as

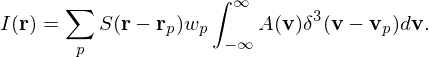∑ ∫ ∞ I(r) = S(r− rp)wp A (v)δ3(v− vp)dv. p −∞

(35)

By using the property of the Dirac delta function, the above equation is written as

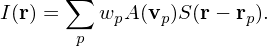∑ I(r) = wpA (vp)S(r− rp). p

(36)