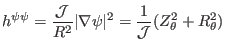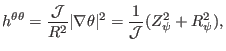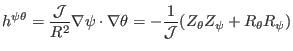To calculate the expression in Eq. (423), we need to calculate the
metric elements of the coordinate system
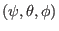 . Note that, in
this case, the coordinate system is
. Note that, in
this case, the coordinate system is
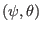 while
while 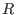 and
and 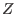 are
functions of
are
functions of  and
and  , i.e.,
, i.e.,
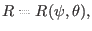 |
(305) |
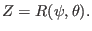 |
(306) |
Next, we express the elements of the metric matrix in terms of 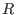 ,
, 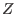 and
their partial derivatives with respect to
and
their partial derivatives with respect to  and
and  . We note that
. We note that
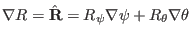 |
(307) |
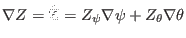 |
(308) |
From Eqs. (307) and (308), we obtain
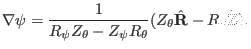 |
(309) |
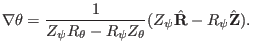 |
(310) |
We note that
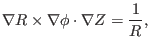 |
(311) |
i.e.,
 |
(312) |
 |
(313) |
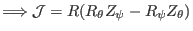 |
(314) |
Using this, Eqs. (307) and (308) are written as
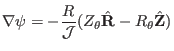 |
(315) |
and
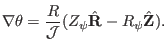 |
(316) |
Using Eqs. (315) and (316), the elements of the metric
matrix are written as
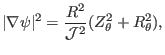 |
(317) |
 |
(318) |
and
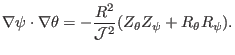 |
(319) |
Eqs. (317), (318), and (319) can be used to
express the elements of the metric matrix in terms of 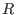 ,
, 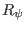 ,
,
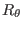 ,
, 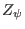 , and
, and
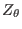 . [Combining the above results, we
obtain
. [Combining the above results, we
obtain
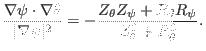 |
(320) |
Equation (319) is used in GTAW code.]
Next, consider the gradient of the generalized toroidal angle  , which
is defined by Eq. (282), i.e.,
, which
is defined by Eq. (282), i.e.,
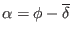 , where
, where
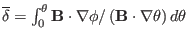 .
The gradient of
.
The gradient of  is written as
is written as
Then
Using the above results,
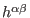 are written as
are written as
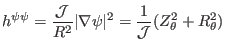 |
(324) |
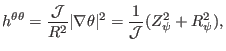 |
(325) |
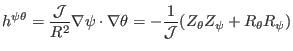 |
(326) |
[As a side product of the above results, we can calculate the arc length in
the poloidal plane along a constant  surface,
surface, 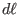 , which is
expressed as
, which is
expressed as
Note that
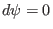 since we are considering the arc length along a
constant
since we are considering the arc length along a
constant  surface in
surface in  plane. Then the above equation is reduced
to
plane. Then the above equation is reduced
to
which agrees with Eq. (188).]
yj
2018-03-09
![]() . Note that, in
this case, the coordinate system is
. Note that, in
this case, the coordinate system is
![]() while
while ![]() and
and ![]() are
functions of
are
functions of ![]() and
and ![]() , i.e.,
, i.e.,
![]() , which
is defined by Eq. (282), i.e.,
, which
is defined by Eq. (282), i.e.,
![]() , where
, where
![]() .
The gradient of
.
The gradient of ![]() is written as
is written as
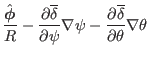

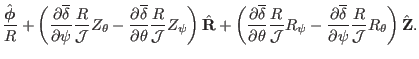
![$\displaystyle \left( - \frac{R}{\mathcal{J}}
Z_{\theta} \hat{\mathbf{R}} + \fra...
...partial
\psi} \frac{R}{\mathcal{J}} R_{\theta} \right) \hat{\mathbf{Z}} \right]$](img1051.png)
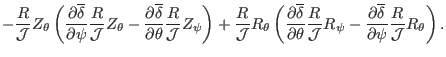
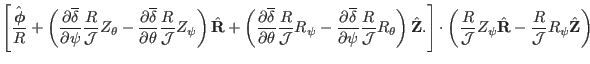
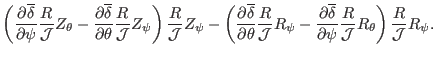
![]() are written as
are written as