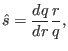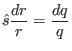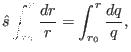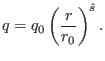The magnetic shear for a concentric circular configuration is defined by
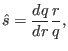 |
(363) |
where 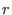 is the minor radius of a magnetic surface. The above expression can
be re-arranged as
is the minor radius of a magnetic surface. The above expression can
be re-arranged as
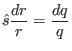 |
(364) |
Integrating the above equation over 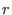 and assuming
and assuming 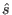 is a constant,
we obtain
is a constant,
we obtain
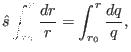 |
(365) |
Performing the integration, the abvoe equation is written as
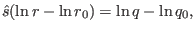 |
(366) |
where
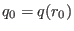 . Equation (366) can be finally written as
. Equation (366) can be finally written as
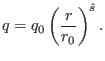 |
(367) |
This is a profile with a constant magnetic shear 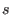 . In Ben's toroidal ITG
simulation, the following
. In Ben's toroidal ITG
simulation, the following 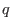 profile is used:
profile is used:
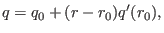 |
(368) |
with
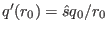 . This is a linear profile over
. This is a linear profile over 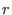 , with
the values of
, with
the values of 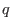 and the shear at
and the shear at 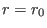 being
being 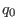 and
and 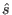 ,
respectively.
,
respectively.
yj
2018-03-09