Laplacian operator in general coordinates (ψ,𝜃,ζ)

6.7 Laplacian operator in general coordinates (ψ,𝜃,ζ)

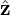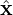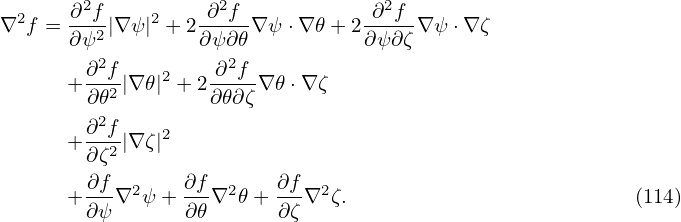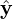
The Laplacian operator is deﬁned by ∇² ≡∇⋅∇. Then ∇²f is written as (f is an arbitrary function)

2 ∇ f = ∇ ⋅∇(f ) = ∇ ⋅ -∂f∇ ψ+ ∂f∇ 𝜃+ ∂f-∇ζ . (111) ∂ ψ ∂𝜃 ∂ζ

To proceed, we can use the divergence formula (110) to express the divergence in the above expression. However, the vector in the above (blue term) is not in the covariant form desired by the divergence formula (110). If we want to directly use the formula (110), we need to transform the vector (blue term in expression (111)) to the covariant form. This process seems to be a little complicated. Therefore, I choose not to use this method. Instead, I try to simplify expression (111) by using basic vector identities:

2 ( ∂f) ( ∂f) (∂f ) ∇ f = ∇ ∂ψ- ⋅∇ ψ+ ∇ ∂𝜃- ⋅∇ 𝜃+ ∇ -∂ζ ⋅∇ζ + ∂f∇2 ψ + ∂f∇2 𝜃+ ∂f-∇2ζ. (112) ∂ψ ∂𝜃 ∂ζ

Using the gradient formula, the above expression is further written as

∂2f ∂2f ∂2f ∇2f = ---2|∇ ψ|2 +-----∇ ψ⋅∇ 𝜃+ -----∇ ψ⋅∇ ζ ∂ψ ∂ψ ∂𝜃 ∂ψ ∂ζ + -∂2f-∇𝜃 ⋅∇ ψ + ∂2f|∇𝜃|2 +-∂2f-∇𝜃 ⋅∇ζ ∂𝜃∂ψ ∂𝜃2 ∂𝜃∂ζ -∂2f- -∂2f-- ∂2f- 2 + ∂ζ∂ψ ∇ζ ⋅∇ψ + ∂ζ∂𝜃∇ ζ ⋅∇ 𝜃+ ∂ ζ2|∇ ζ| ∂f ∂f ∂f + ∂ψ-∇2ψ + ∂𝜃∇2 𝜃+ ∂ζ-∇2ζ, (113)

and can be simpliﬁed as

∂2f ∂2f ∂2f ∇2f = --2|∇ψ |2 + 2----∇ ψ⋅∇ 𝜃+ 2-----∇ ψ⋅∇ ζ ∂ψ2 ∂ ψ∂2𝜃 ∂ ψ∂ζ + ∂-f|∇ 𝜃|2 + 2-∂-f-∇𝜃 ⋅∇ζ ∂𝜃2 ∂𝜃∂ ζ ∂2f- 2 + ∂ζ2|∇ ζ| ∂f 2 ∂f 2 ∂f 2 + ∂ψ-∇ ψ + ∂𝜃∇ 𝜃+ -∂ζ∇ ζ. (114)

Assume (ψ,𝜃,ζ) are ﬁeld-line following coordinates with ∂r∕∂𝜃 along the ﬁeld line direction, then neglect all the parallel derivatives, i.e., derivative over 𝜃, then the above expression is reduced to

∇2f = ∂2f|∇ ψ|2 + 2 ∂2f-∇ ψ ⋅∇ζ + ∂2f|∇ζ|2 ∂ψ2 ∂ψ∂ζ ∂ζ2 ∂f- 2 ∂f- 2 +∂ ψ∇ ψ + ∂ ζ∇ ζ. (115)

This approximation reduces the Laplacian operator from being three-dimensional to being two-dimensional. This approximation is often called the high-n approximation, where n is the toroidal mode number (mode number along ζ direction).

[next] [prev] [prev-tail] [front] [up]