A.16 (s,α) parameters

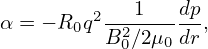
The normalized pressure gradient, α, which appears frequently in tokamak literature, is defined by[3]

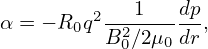(622)

which can be further written

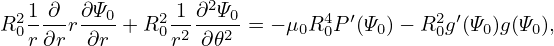(623)

where p = p∕(B₀²∕2μ₀). Equation (623) can be further written as

(624)

where 𝜀_a = a∕R₀, r = r∕a, and a is the minor radius of the boundary flux surface. (Why is there a q² factor in the definition of α?)

The global magnetic shear s is defined by

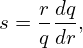(625)

which can be written

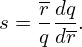(626)

In the case of large aspect ratio and circular flux surface, the leading order equation of the Grad-Shafranov equation in (r,𝜃) coordinates is written

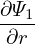(627)

which gives concentric circular flux surfaces centered at (R = R₀,Z = 0). Assume that J_ϕ is uniform distributed, i.e., |J_ϕ| = I∕(πa²), where I is the total current within the flux surface r = a. Further assume the current is in the opposite direction of ∇ϕ, then J_ϕ = −I∕(πa²). Using this, Eq. (627) can be solved to give

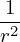(628)

Then it follows that the normalized radial coordinate ρ ≡ (Ψ − Ψ₀)∕(Ψ_b − Ψ₀) relates to r by r = 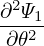 (I check this numerically for the case of EAST discharge #38300). Sine in my code, the radial coordinate is Ψ, I need to transform the derivative with respect to r to one with respect to Ψ, which gives

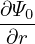(629)

(630)

The necessary condition for the existence of TAEs with frequency near the upper tip of the gap is given by[3]

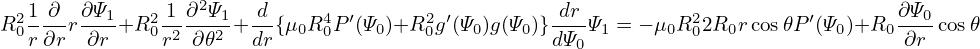(631)

which is used in my paper on Alfvén eigenmodes on EAST tokamak[15]. Equations (629) and (630) are used in the GTAW code to calculate s and α.